Category:Tensors

**tensor**
	multilinear map on some combination of scalars, vectors, covectors, and tensors
	Upload media
	Wikipedia Wikiversity
Instance of	geometric concept; general quantity;
Subclass of	multilinear map; mathematical object;
Part of	tensor space;
Different from	Q15990710; tensor quantity;
Authority file
	Q188524; NDL Authority ID: 00572865; BNCF Thesaurus ID: 31131
	Reasonator; Scholia; Wikidocumentaries; PetScan; statistics; WikiMap; Locator tool; KML file; Search depicted;

<nowiki>tensor; Tensor; 텐소르; Тензор; Tensor; تەنزور; Тензор; tensor; 張量; Tenzor; тензор; 張量; 张量; tenzor; Тензор; tensoro; tenzor; টেন্সর; tenseur; Tenzor; tenxơ; تەنزور; Tenzor; тензор; tensor; 张量; Тензор; tensor; tensor; Tenzor; tensor; موتر; တာအုံ; 張量; Tenzor; Tensore; Cálculu tensorial; tensor; Tensor; Tensor; Trajtimi klasik i tensorëve; Тензор; 張量; テンソル; טנזור; Тензор; आतानक विश्लेषण; 张量; ਟੈਂਸਰ; Tensor; Tenzor; 张量; tensore; 張量; тензор; teinseoir; Tensor; tensor; tensor; nacrtensori; تانسور; tensori; tinsuri; tensor; տենզոր; Tenzor; тензор; Tenzorius; tenzor; Tensor; тензор; tenzor; tensor; tensor; ടെൻസർ വിശ്ലേഷണം; tensor; tensor; tensör; тэнзар; 텐서; tensor; тензор; Τανυστής; Tenzors; objeto algebraico; objet très général dont la valeur s'exprime dans un espace vectoriel; геамэтрычны аб’ект; понятие в математике и физике; multilineare Abbildung; transformação multilinear em alguma combinação de escalares, vetores, covetores e tensores; մաթեմատիկական և ֆիզիկական հասկացություն; 數學物件; вектор одређеног векторског простора, вредности зависе од координата, а математички се предствљају матрицама; skaler, vektör, covector ve tensörlerin bazı kombinasyonlarında çok çizgili harita; 線形的な量または線形的な幾何概念を一般化したもの; transformação multilinear em alguma combinação de escalares, vetores, covetores e tensores; objek geometris, untuk memetakan suatu objek geometris ke objek lainnya; uogólnienie pojęcia wektora; geometrisk objekt; applicazzioni multiliniari ca associa un nùmmiru dûn campu ô nsiemi di na siquenza di vitturi cafannu parti di nu spazziu vitturiali supra a ddu campu e di na siquenza di covitturi ca fannu parti dû spazziu duali di ddu spazziu vitturiali; 多重线性映射; 多重线性映射; matematiikassa tietyn tyyppinen geometrinen kokonaisuus tai vaihtoehtoisesti yleinen suure; multilinear map on some combination of scalars, vectors, covectors, and tensors; mapa multilinear d'alguna combinació d'escalars, de vectors, de covectors i de tensors; v matematice objekt, který je zobecněním pojmu vektor; generalizzazione delle strutture definite usualmente in algebra lineare a partire da un singolo spazio vettoriale; Tenzorska enačba; テンソルの次数; tensorfält; tensorer; tensörler; çok çizgili harita; חשבון טנזורים; טנסור; tensor (mathematica); 張量的經典處理; 張量的中間處理; टेंसर; 张量; تنسور; تينسور; موتّر; ممتد; مسرد نظرية التينسور; 张量的经典处理; 张量的中间处理; حساب تانسوری; حساب تانسور; ثابت کیهان‌شناسی; 张量的经典处理; 张量的中间处理; тензори</nowiki>

English: In mathematics, a tensor is a certain kind of geometrical entity and array concept. It generalizes the concepts of scalar, vector and linear operator, in a way that is independent of any chosen frame of reference. For example, doing rotations over axis does not affect at all the properties of tensors, if a transformation law is followed. Tensors are of importance in pure and applied mathematics, physics and engineering.

Subcategories

This category has the following 10 subcategories, out of 10 total.

F

Tensor fields (4 C, 1 P, 17 F)

M

Multivectors (3 C, 11 F)

P

Pyramids of numbers (2 C, 1 F)

S

Stress (mechanics) (26 C, 74 F)

Media in category "Tensors"

The following 51 files are in this category, out of 51 total.

2Tensor representation 2D or 3D.png 506 × 206; 24 KB
Another universal tensor prod.svg 248 × 142; 19 KB
Bacterial-tensor-product.svg 512 × 181; 14 KB
Bacterial-tensor-twice-contracted-product.png 548 × 151; 243 KB
Calcul tensoriel-fr.pdf 1,239 × 1,754, 27 pages; 1.05 MB
Contravariant and covariant components of a vector.jpg 583 × 448; 18 KB
Covariance and contravariance of vectors.jpg 519 × 689; 28 KB
Covariant vector under coordinate transformation.svg 531 × 530; 79 KB
Cube, Vector of Cubes, Matrix of Cubes, Cube of Cubes.png 1,155 × 335; 22 KB
Curve3D.png 560 × 420; 22 KB
Curve3DST.png 560 × 420; 3 KB
De-Tensor.ogg 1.6 s; 15 KB
Decomposition of tensor components.svg 1,134 × 602; 64 KB
Definizione Prodotto Tensoriale.svg 133 × 61; 13 KB
Epsilon volume anticyclic permutations.svg 484 × 558; 64 KB
Epsilon volume cyclic permutations.svg 445 × 588; 62 KB
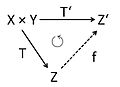
Factorisation property of the tensor product.svg 751 × 626; 21 KB
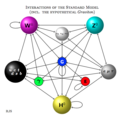
Fundamental Interactions.png 750 × 735; 138 KB
Gautam Number Gn.svg 816 × 1,056; 16 KB
Matrice cube 1.JPG 305 × 300; 28 KB
Matrix tensor.JPG 283 × 200; 12 KB
MatrixTensor.JPG 262 × 171; 12 KB
Notazione tensoriale cartesiana.png 392 × 332; 7 KB
Plasticity models meridional profile.svg 720 × 540; 51 KB
STball.png 560 × 420; 17 KB
STcurvel.png 560 × 420; 18 KB
STgeneric.png 560 × 420; 9 KB
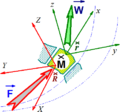
Stress components in element.png 535 × 473; 18 KB
Stress energy tensor of Kartazion.png 571 × 337; 34 KB
STsurfel.png 560 × 420; 10 KB
Tenseurs - exemples.png 631 × 245; 34 KB
Tensor 0.png 438 × 407; 8 KB
Tensor 01.png 490 × 370; 12 KB
Tensor 02.png 607 × 410; 15 KB
Tensor components explained.svg 154 × 1,248; 202 KB
Tensor network contraction example.png 1,906 × 2,380; 142 KB
Tensor of rank two.svg 436 × 244; 11 KB
Tensor Product - Bernstein basis polynomials.svg 958 × 954; 1.45 MB
Tensor Product Construction.svg 228 × 62; 24 KB
Tensor product of algebras.png 392 × 307; 13 KB
Tensor product1.gif 250 × 100; 2 KB
Tensor product2.gif 250 × 100; 2 KB
Tensor train.png 1,253 × 122; 12 KB
Tensor-diagram.jpg 684 × 496; 26 KB
Tensor-diagram.svg 190 × 104; 15 KB
Tensor-diagramB.jpg 684 × 496; 18 KB
Tensor-order-comparison.png 567 × 208; 16 KB
Tensorbild.svg 256 × 210; 30 KB
Tenzor1.png 283 × 198; 1 KB
Tenzor3.png 482 × 255; 2 KB
UnicitaPT.gif 324 × 191; 2 KB

Category:Tensors

Subcategories

*

B

C

D

F

M

P

S

Media in category "Tensors"

Navigation menu

Search